Boulos El Hilany
Research Interests
Polynomial systems and maps
Complex, and real, polynomial equations appear as polynomial systems, or maps, and encode a topological structure that is crucial to understand in order to answer questions in pure, and mathematics applied to sciences and engineering. Such polynomials retain distinguished discrete structures, making its exploitation important for the optimality of computational processes.
In this direction, my research concerns creating, and employing procedures to describe the resulting topologies by taking advantage of polynomials' structure and geometry.
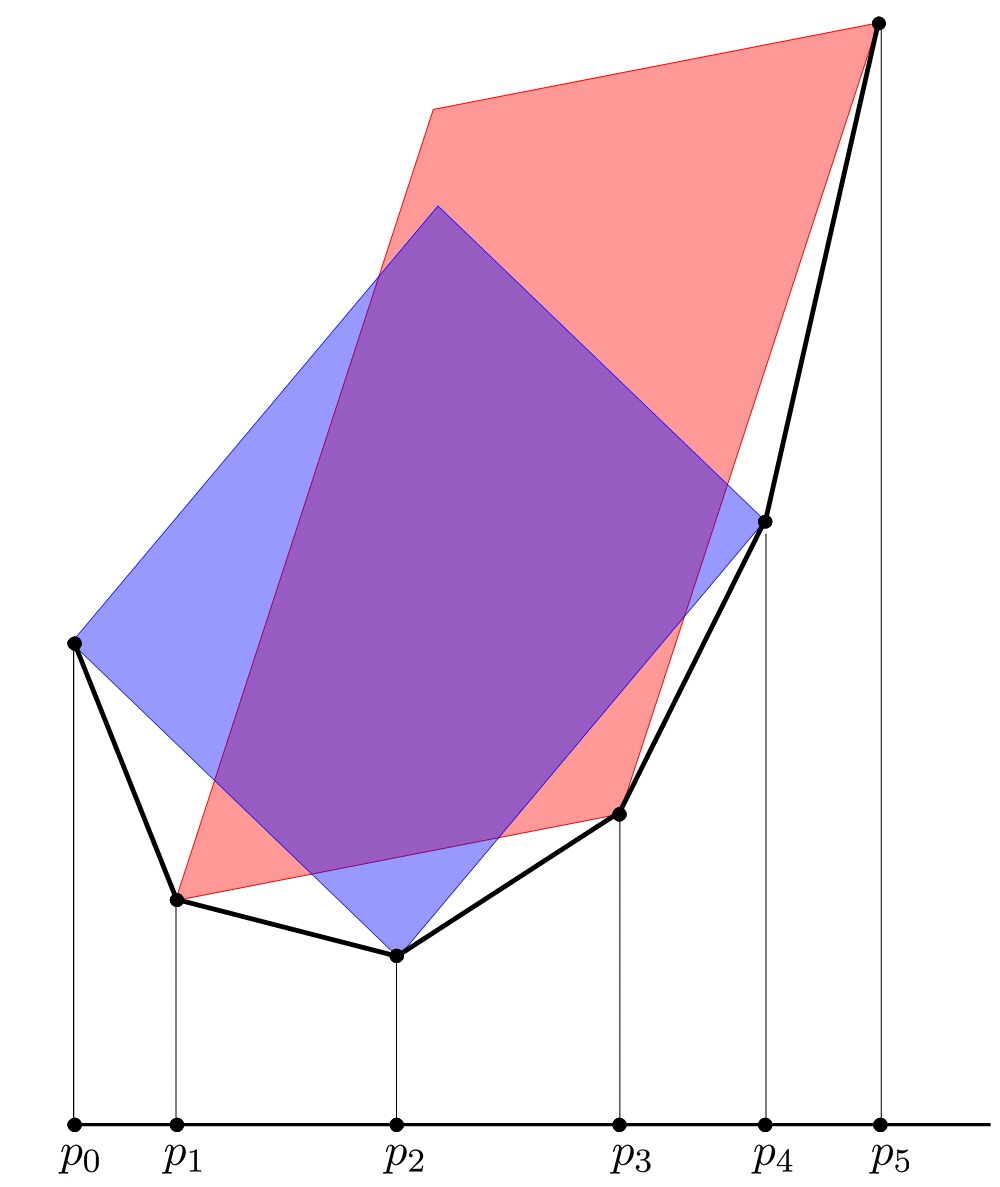
Fewnomial theory:
Computing the real isolated solutions to a square system of polynomial equations is a substantially difficult problem. One thus tries to estimate their number by providing upper bounds that depend on the number of existing monomial terms. Additionnally, constructing polynomial maps with many real solutions has the purpose of understanding known upper bounds' optimality, and surpisingly little such constructions have been made.
Examples of tools I use to improve known upper bounds, and test sharpness include:
- Combinatorial patchworking
- A-discriminant varieties
- Dessins d'enfant
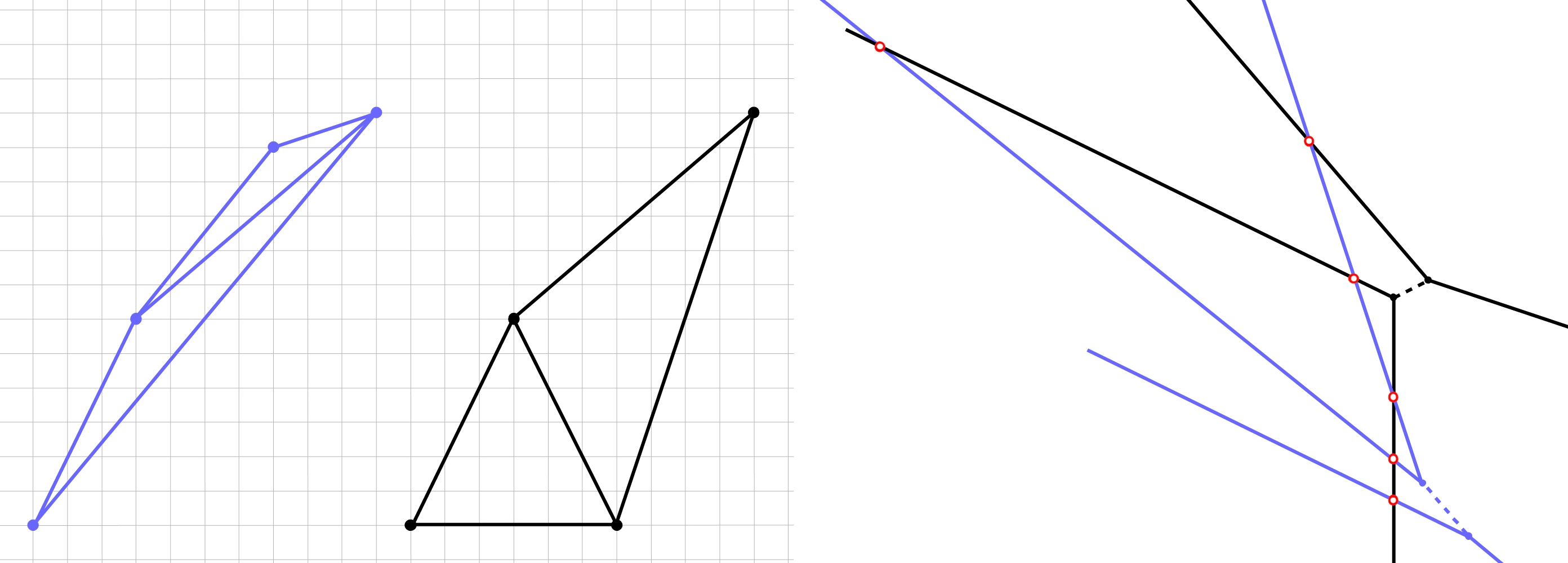
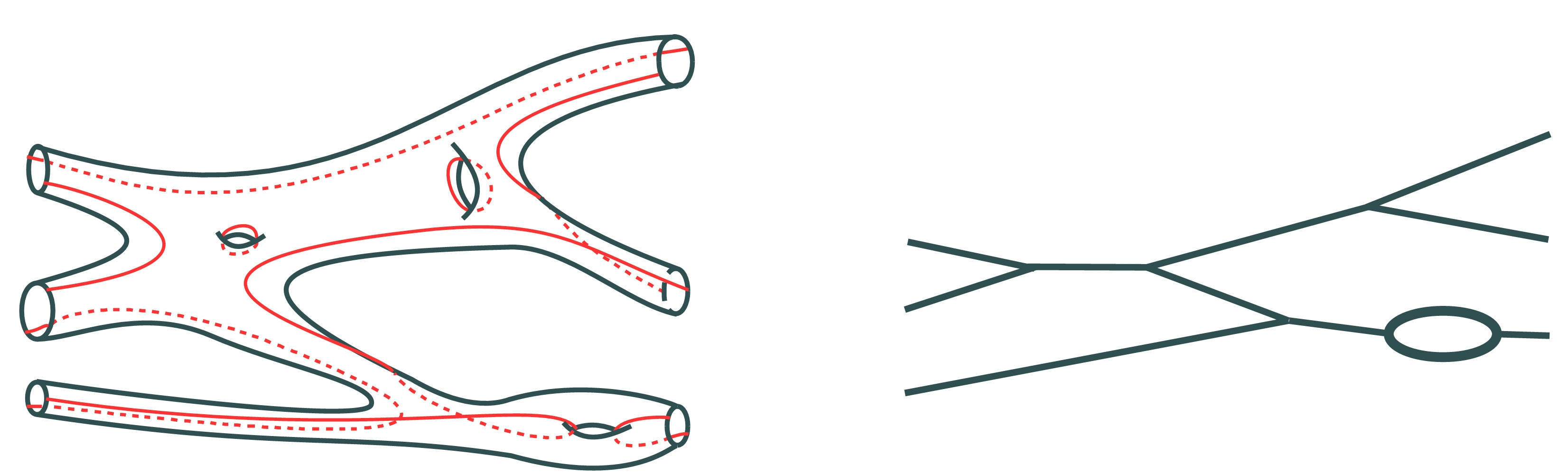
Topology of polynomial maps:
The customary problem regarding polynomial maps from one complex (or real) space to another is to have a clear topological picture of the values at which the map admits an "atypical" topology. These values form a locus called the bifurcation set, whose properties are mysterious, and equations are hard to reach. The deficiency of knowledge about this object hinders much progress in answering questions regarding:
- The relation between topology of maps, and structure of polynomials
- Classification of topological types of polynomial maps
- Description of polynomial maps having extremal topology
Tools I use to describe the bifurcation set:
- Polyhedral geometry of polytopes
- A-discriminant varieties
- Tropical geometry
-
Toric geometry
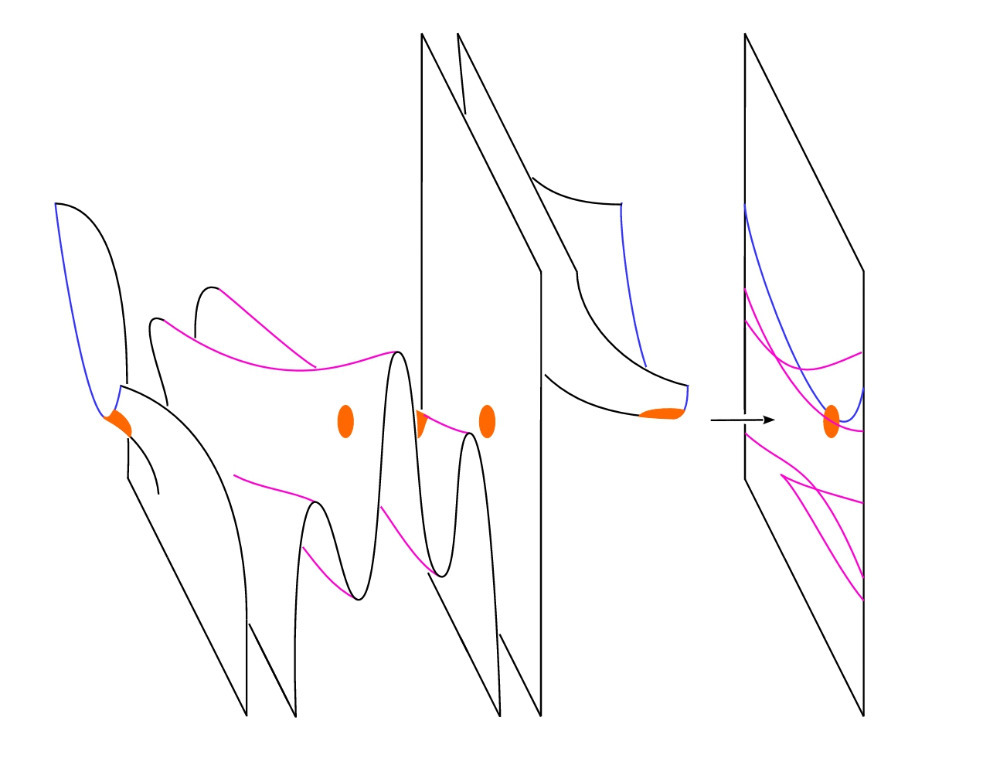
Real Hurwitz numbers
Hurwitz numbers count isomorphism classes (i.e. up to homeomorphisms) of smooth, compact Riemann surfaces which are ramified covers of the complex projective line, with given data over a set of branch points in the target. Such counts have important connections to classical problems such as the moduli space of curves, and matrix models in probability theory. This enumeration turned out to be a hard problem with no universal recipe.
Consider now the above problem, but modified so that to include a real structure (i.e. analogue of the complex conjugation) on the ramified cover. This constraint makes the problem dependent on the the critical value's position, thus augmenting the problem's difficulty. Hence, one aims to provide non-trivial lower bounds by defining a signed count for covers, and showing that it is independent of this extra datum.
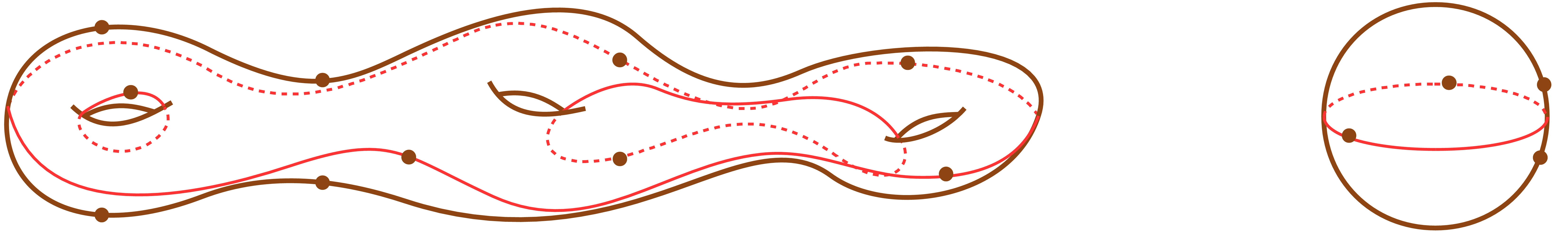
Tools for studying real Hurwitz covers:
- Real dessins d'enfan
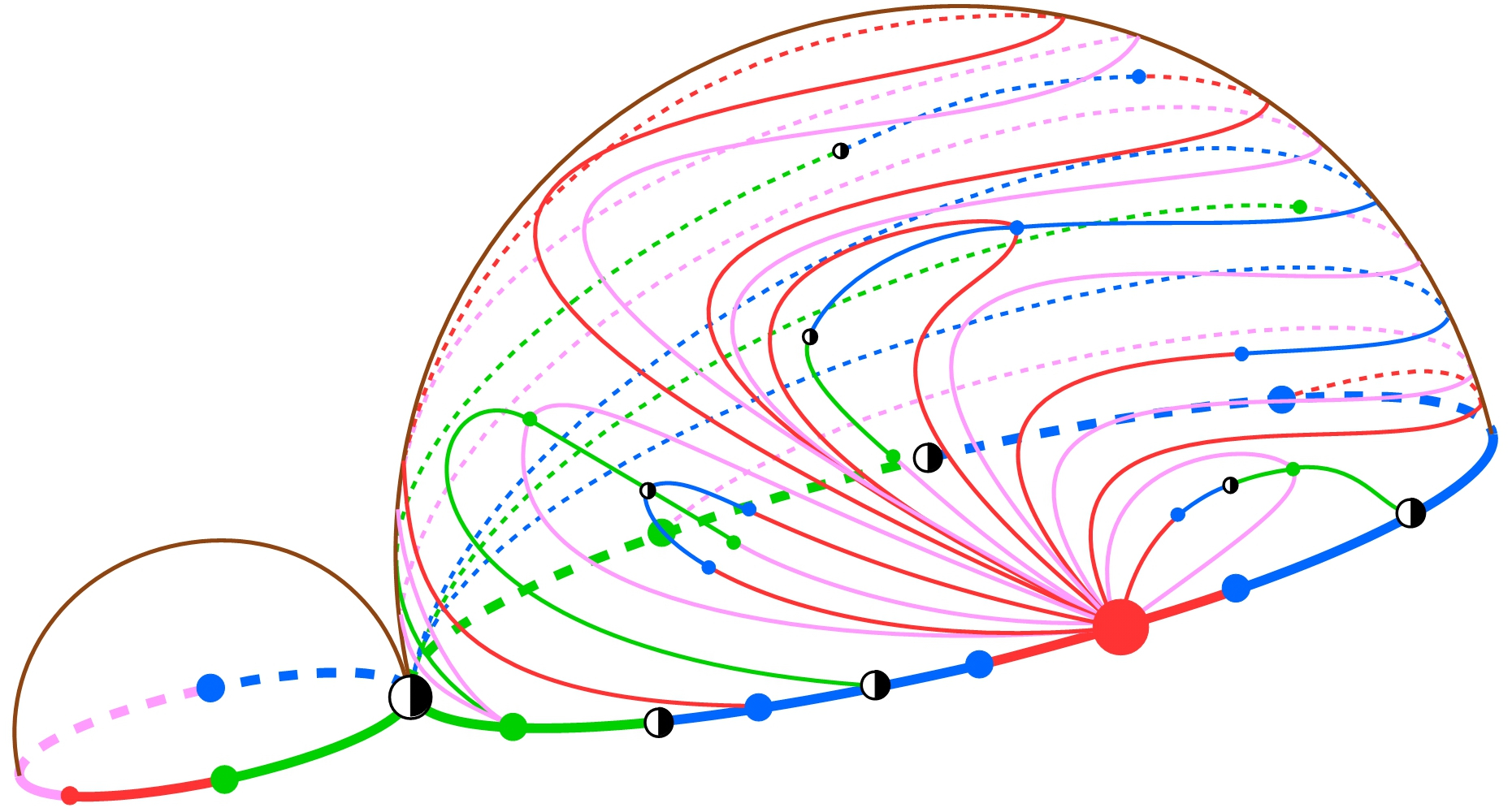
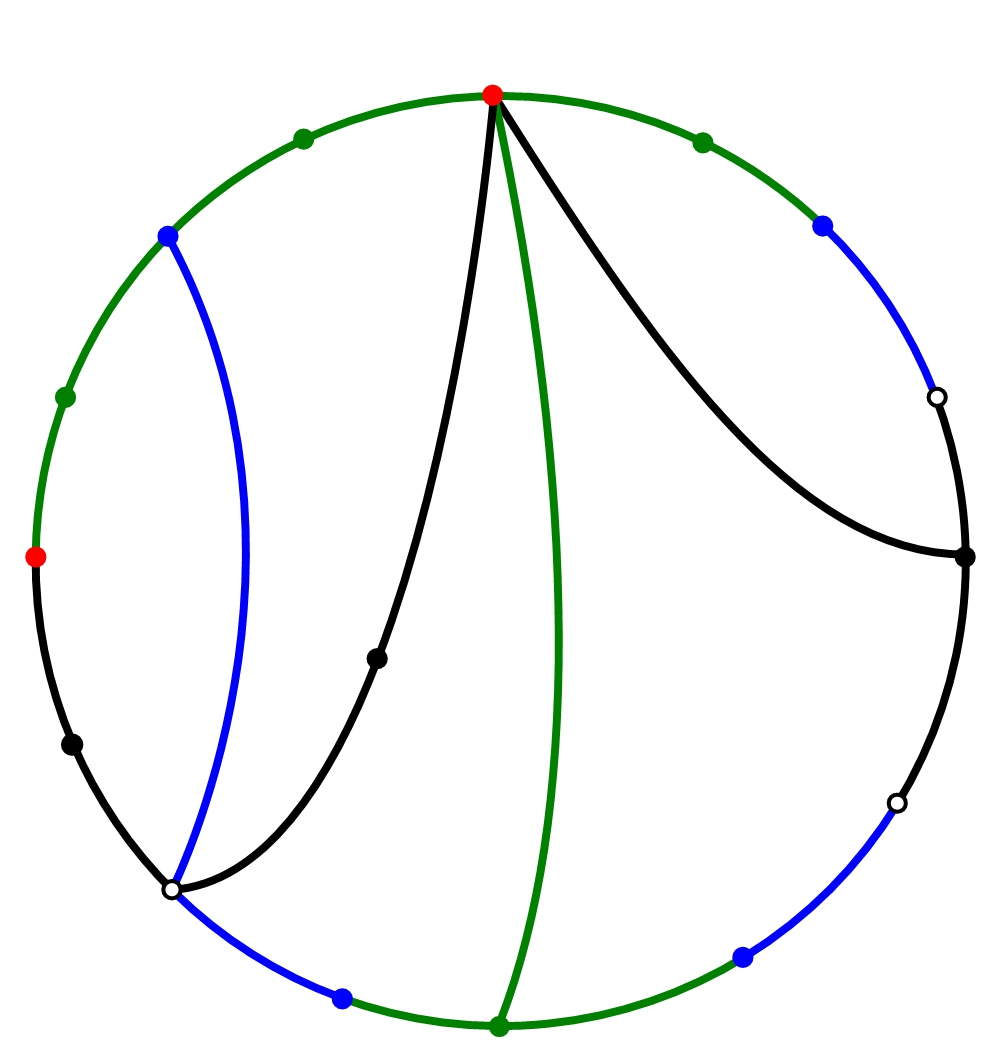
- Tropical Hurwitz covers